Toward robust proton therapy planning and delivery

With its significant advantages of physical dose deposition, specifically, the ability to select the energy of a proton beam such that it stops in patient at a known depth, thereby depositing no dose in tissue distal to the target, proton therapy is gaining wider acceptance as an additional modality in radiation therapy treatments. Many uncertainties, however, are associated with the clinical application of proton therapy, including calculation of beam range in patient from patient CT images; uncertainties in beam lateral scattering and penumbra values in patient; sensitivity to patient set up error and intra-fraction organ motion; and sensitivity to patient anatomical and physiological changes through the course of treatment, including tumor shrinkage and organ filling variations in stomach and bowels. Many studies have been performed to quantify and evaluate the dosimetric effects of such uncertainties. In the clinical practice of proton therapy, every effort should be made in the patient simulation, planning, and delivery process to take these uncertainties into consideration, such that proton treatments are delivered with adequate robustness and high confidence against their effects.

Uncertainty of CT number to relative stopping power ratio (RSPR) conversion

For each treatment field, the beam range required to adequately cover the target distally is calculated from the water-equivalent-path-length (WEPL) of the beam, from the location where it first enters the patient to the distal most point of the target. CT numbers of patient anatomy along the beam path is converted to tissue-to-water relative stopping power ratio (RSPR) of protons, using a pre-determined CT number conversion curve, such as the stoichiometric method of Schneider et al. (1). Uncertainties in the determination of such conversion curves, including accuracy of calculated RSPR of tissue-equivalent materials used to establish such curves (2); CT imaging beam hardening effect (3); and the weak dependence of CT numbers on tissue compositions; translate directly into uncertainties in beam range calculation. Clinically, proximal and distal margins of up to 3.5% have been added to the calculated beam range to accommodate for such uncertainties (4) see Figure 1. Schaffer and Pedroni (3) performed animal tissue stopping power measurements in comparison with the calculated values using the stoichiometric method, and found agreements to within 1.1% in soft tissues and 1.8% in bones, with CT beam hardening contributing to less than 1% of the uncertainties. Yang et al. (5) performed a comprehensive analysis of proton beam range uncertainties in the CT number to relative stopping power ratio conversion using the stoichiometric method. It was noted that uncertainties associated with the calculated RSPR values are highly dependent on tissue groups, ranging from 1.6% to 5.0%, with smaller values of uncertainties for soft tissues and higher values for higher-density tissue groups such as bones. The overall uncertainties of beam range determination, for most clinical disease sites, were estimated at 3.0-3.4% at 95 percentile confidence interval.

Figure 1 Use of proximal and distal margins to account for CT number to RSPR conversion uncertainties: a distal margin is included in beam range selection to assure distal CTV coverage in the event that the calculated beam range overestimates the delivered beam range; while a proximal margin is used to assure proximal CTV coverage in the event that the calculated beam range underestimates the delivered beam range.

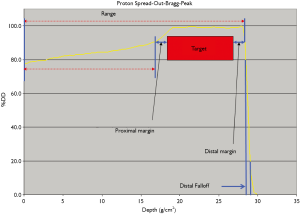
It should be noted that use of proximal and distal margins to accommodate for CT number to RSPR conversion uncertainties assumes that target coverage by the prescribed isodose distribution is prioritized at distal end of the beam: addition of a distal margin guarantees target coverage in the event that the delivered beam has a range in patient shorter than calculated from the CT number to RSPR conversion curve; while use of a proximal margin assures target coverage in the event that the delivered beam range is larger than calculated. In the case where delivered beam range is larger than calculated beam range, beam penetration is deeper than shown in a treatment planning system, therefore delivering a prescribed dose to an additional thickness of tissue located distal to the target than calculated by the treatment planning system. A critical organ located distal to the target may therefore receive the full prescription dose by use of a distal margin to the beam. For this reason, it is generally recommended that proton beam angles are selected to avoid having a critical organ immediately distal to the target. Proximal margins should also be adequate to assure target coverage for the case where delivered beam range is larger than calculated, especially for beams that enter target through low density tissues such as the lung (6). In addition, verification treatment plans, using the same beam range and modulation values, may be re-calculated using alternative CT number to RSPR conversion curves that are scaled up or down by the expected uncertainty limits (for example +/-3.5%), to investigate the cumulative effect of such uncertainties in terms of both target dose coverage and critical organ doses, and the original treatment plan modified if unacceptable target and critical organ doses were identified in the verification plans.

While proximal and distal margins have been the method of choice to account for CT number to RSPR conversion uncertainties for traditional, scattered broad beam proton therapy techniques, their implementation for intensity modulated proton therapy techniques using pencil or spot beam scanning techniques is not as well-established. In particular, the delivered dose distribution degradations, in terms of both dose inhomogeneities and target coverage, due to potential misplacement of individual beam spots, are not completely resolved by use of distal and proximal margins, as shown by Albertini et al. (7). While effect of range uncertainties may be estimated by recalculating treatment plans using CT number to RSPR conversion curves scaled up to down a given percentage (8), complete integration of such uncertainties in IMPT optimization algorithms remains unavailable in commercial treatment planning systems. Inaniwa et al. (9) proposed an IMPT optimization algorithm that allows adequate target coverage while maintaining allowable dose distribution gradients within the target. Liu et al. (10) developed an IMPT optimization algorithm based on worst-case dose distributions that includes setup errors as well as range uncertainty. A total of nine different dose distributions that include the effects of these uncertainties are calculated each iteration of plan optimization, and optimization objective function evaluated based on the worst dose distribution of the 9 plans. The authors found that the proposed algorithm able to improve normal tissue sparing while maintaining plan robustness against the uncertainties considered, without using the “safety margins” of traditional PTV concept.

Dosimetric effect of setup errors and organ motion

In general, patient setup errors and organ motion tend to cause geometric misalignment of treatment field to the treatment target [clinical target volume (CTV)] in radiotherapy, resulting in part of the CTV receiving inadequate dose coverage. Traditionally, these uncertainties are compensated for in treatment planning by use of the Internal Target Volume (ITV) and Planning Target Volume (PTV) concept (11), which expand the treatment field laterally in the beam’s eye view (BEV) such that the CTV or ITV is always contained within the treatment field. In proton therapy treatments, however, patient setup errors and organ motion not only affect the lateral extent of proton dose distribution, but also cause uncertainties in beam range within the treatment field. In that sense, the traditional PTV expansion, using magnitude of target motion and setup errors to calculate expansion margins, does not in principle apply to proton therapy. A setup error parallel to beam direction, if not associated with a corresponding WEPL change, has minimal effect on proton beam dose distribution, thus may be safely ignored in proton therapy treatment planning.

A state-of-the-art proton therapy treatment planning system for scattered and/or or uniform scanning (wobbling) proton beam dose calculations, using the pencil beam dose calculation algorithm (12,13), decomposes a broad, scattered proton beam into small pencil beamlets, and performs ray-tracing of each proton pencil beam to determine the WEPL of the pencil required for the treatment field. A range compensator (or “bolus”) is used to pull back the ranges of individual pencil beams so that the board beam conforms distally to the treatment target (14,15). Patient setup errors, both translational and rotational, may change the calculated WEPL values across the treatment field, for example, increasing the WEPL of a pencil beamlet required to reach the distal side of the target if a higher density bone, absent in the path of a beamlet in the treatment planning images, moves into the path of the beamlet due to setup errors. Figure 2 from Urie et al. (15) illustrates how the range compensator is “smeared” (expanded) to account for the WEPL changes of setup errors and organ motion on a given beamlet. The tissue-equivalent range compensator is calculated using ray tracing for each beamlet, and has variable thicknesses across its profile so that all beamlets would stop at the distal surface of the target. In regions where the beam passes through high density bone segments, the range compensator has reduced thickness due to the increased WEPL through the bone (for example, line from points P through S to Td). If the bone is offset from the range compensator laterally due to patient setup errors or organ motion, then a beamlet may pass though thicker part of the compensator, as well as the bone segment, causing it to stop before reaching the distal surface of the target. The thinner part of the range compensator is therefore smeared by a given radius, called “smearing margin”, the magnitude of which should be no smaller than the potential total shift of the bone relative to the compensator due to setup errors and/or organ motion (d in Figure 2).

Figure 2 Modification of range compensator to account for effect of beamlet range changes due to setup errors. (A). Sample ray line along which a line integral is performed to obtain the WEPL between skin (S) and the proximal (Tp) and distal (Td) target surfaces. (B). Expansion (smearing) of range compensator to ensure target coverage in the presence of setup error and organ motion of magnitude d. From (15).

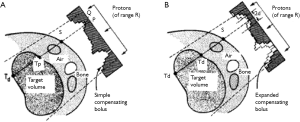
As discussed above, range compensator design is based on geometric ray tracing of the WEPL values of beamlets within the treatment field to the distal surface of the target. This method of compensator design, however, does not adequately consider the additional scattering and range straggling of protons passing through the compensator itself. Range compensators are therefore fabricated with a tapered milling bit on a CNC milling machine, in order to introduce an tapering angle (up to 3^o) that reduces the effect of protons scattering off sharp compensator edges and the resulting dose distribution inhomogeneities (hot-spots) (14). Range compensators may also be “smoothed” manually by interpolation of its thicknesses for beamlets into final grids for fabrication. This practice has been found to improve agreement of calculated vs. measured dose distribution, and in general improves dose distribution homogeneity (16). The effectiveness of compensator smearing is also reduced by the additional proton range straggling introduced by the compensator. To include the effect of such range straggling, Moyers et al. (4) included an additional term of proton lateral scattering term in their smearing margin (referred to as “bolus expansion (BE)” in the article) calculation, to arrive at an equation of

BE=(IM+SM)2+0.03×(distalCTVdepth+bolusthickness)2,

where IM is the internal organ motion radius in the direction orthogonal to beam axis, and SM is the setup error term, again in the direction orthogonal to beam axis.

The borders of the compensator which overlaps with the additional PTV and aperture margins of the treatment field will not produce intersections with the target in ray tracing. Thicknesses of the compensator in these parts are usually set to be equal to the compensator thicknesses immediately inside the target in the beam’s eye view (BEV), in a practice commonly referred to as “border smoothing”. The radius of border smoothing should therefore be selected to be no smaller than the margins between the field border and the target.

Adequate selection of range compensator smearing and border smoothing margin values in general assures adequate target coverage of scattered proton beam treatments. This method of increasing proton beamlet ranges to assure adequate distal target coverage, however, results in unnecessary treatment of normal tissues immediately deeper than the target for the times when the higher density tissues do not intersect the beamlets for which the smearing margin is selected. Use of smearing margins, therefore, may increase doses to critical organs located immediately distal to the target. Similar to the case of range uncertainties discussed previously, careful considerations should be given to beam angle selection to avoid such scenarios.

Calculation of proximal and distal margins, as outlined above, assumes knowledge of magnitudes of organ motion and setup errors for each patient. Magnitude of periodic organ motion, such as due to breathing, is available via use of 4-dimensional CT (4DCT) or MRI imaging techniques. Use of such data however needs to be cautioned with the understanding that their reproducibility throughout the course of a patient’s radiation therapy treatment is not assured (17). Periodic repeat 4DCT scans during a patient’s treatment course may be necessary to confirm the consistency of such motion data. Non-periodic organ motion, such as prostate movement due to bladder filling and rectal gases, may require separate and additional and sometimes patient-specific evaluation.

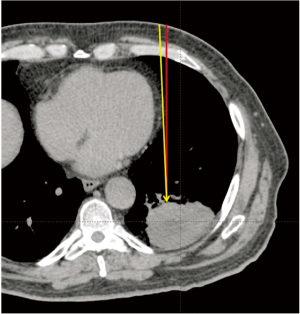
It should be further noted that the setup errors that have impact on selection of proximal and distal margins, as well as smearing margins in range compensator design, are heavily influenced by multiple factors. While in traditional photon therapy one would be concerned only with the setup error of the target itself, for proton therapy one is concerned with the setup errors of the target, as well as all tissues that lie in the path between beam entrance and the distal target, for each individual beam. Patient skin and the underlying fat/muscle tissue may not be reproducible, especially when compressed by immobilization devices. Bony structures that a beam traverses through may present different angulations between treatment fractions. Rotational setup errors, both for overall patient anatomy, as well as internal organs, such as bones and, in the thorax, the mediastinum and the heart, can cause large range errors. Figure 3 shows the potential effect of rotational setup error: if an anterior-posterior (AP) beam is used for treating this lung cancer, a rotational error of 2 degrees in patient roll direction would place the beam path though a thickness of the heart (yellow line) that was not present in the planned beam path (red line). The heart tissue in the beam path causes an increase of 2.2 cm in WEPL required to reach the target. Such a sharp change in WEPL values could be compensated for by use of range compensator smearing margins, which nonetheless increases dose distribution heterogeneity. Note that this type of changes may not be due to setup errors, but can be the result of organ motion: breathing motion, as well as heart beating, can cause rotation of the heart by greater than 2 degrees. It is therefore recommended that such treatment beams be avoided in proton therapy treatment plans.

Figure 3 Effect of rotation setup errors on the range of a proton beam tangential to the heart. The planned beam path, shown in red, has a WEPL value of 8.48 cm to reach the tumor. With a setup error of 2 degrees, the altered beam path, shown in yellow, has a WEPL value of 10.68 cm. This 2 degree setup error in patient roll direction therefore introduces a beam range uncertainty up to 2.2 cm.

Trofimov et al. (18) performed detailed analysis of the effect of setup errors in the treatment of prostate cancer using proton therapy. As proton therapy fields in prostate cancer treatments typically use lateral beam entrance gantry angles, femur rotation angles, as well as thicknesses of subcutaneous tissue, in addition to prostate position and rotation, all contribute to uncertainties in the delivered proton dose distributions. The authors found substantial variations in the software tissue thicknesses in the lateral hips (up to 5 mm), and femur rotation angle deviations from initial values of greater than 10^o. Selection of distal and proximal margins for beam range calculations, as well as smearing margin for the range compensator, needs to take these variations into consideration. Patient immobilization technique and daily setup tolerances, as calculated by image guidance system, should be optimized to minimize such variations. For example, the commonly used vacuum bags in lung cancer treatments can introduce over 10 mm variations in the thicknesses of soft tissue around the chest wall; and breast position in female patients can introduce additional thickness variations. It is therefore common that no such vacuum bag immobilization devices are used for treatment of tumors in the thorax region using proton therapy, and beams that enter female patient through breasts are minimally used.

While the dosimetric effect of organ motion has been traditionally accounted for by use of the ITV concept in photon-based radiation therapy, this practice is often inadequate for proton therapy, especially when the organ motion is accompanied by tissue density changes, such as in the treatment of isolated lung tumors. The solid tumor, often having near tissue RSPR value, is enclosed within low density lung tissue. The ITV volume therefore includes volumes of both low density lung tissue and higher density tumor tissue. Calculation of proton beam range that ensures adequate tumor coverage regardless where the tumor is needs to take this motion-induced RSPR change into consideration. In addition, the normal lung volume has variable RSPR values at different phases of breathing, due to the filling of lung airways and blood vessels. Several authors have described treatment planning strategies for lung cancer (4,19-21). A typical strategy would start with constructing an average CT dataset from the 10 phases of a 4DCT scan set. The ITV is then outlined on the average CT dataset, and its HU values overridden by the maximum or a representative HU value of the tumor. Beam range calculations, as well as range compensator design, is performed on this CT dataset, with appropriate proximal, distal, and compensator smearing margins applied. Note that this approach accounts for the range uncertainties caused by lung density variations in breathing, as well as ITV density variations due to tumor motion. However, the critical organ doses calculated from this CT dataset may be underestimated. A second treatment plan is therefore calculated, using the same beam parameters (apertures, compensators, beam ranges, beam modulation widths, etc.) of the initial treatment plan, but using an average CT dataset with no override of ITV HU values. Finally, several verification plans of the initial plan may be calculated on representative phases of 4DCT images (for example maximum inhalation, mid-inhalation, and maximum exhalation phases) to confirm the adequacy of target coverage as well as critical organ protection. Treatment planning of proton lung therapy therefore calls for calculation of anywhere between 2 and 5 treatment plans, a significant increase in complexity and workload from the practice of photon-based lung radiotherapy practice.

Detailed and comprehensive consideration of the dosimetric effect of patient setup errors and organ motion is critical in the optimal design of proton therapy treatment plans. It is also a labor-intensive and time-consuming process, as state-of-the-art proton therapy treatment planning systems do not include automatic tools for this process. Much research efforts have been devoted to development of methodologies to perform this task, with greater attention paid to intensity-modulated proton therapy (IMPT) treatment planning (10,22-26). For example, Park et al. (27) proposed the use of beam-specific PTV (bsPTV) to account for setup errors, CT number to RSPR conversion uncertainties, as well as range uncertainties due to organ motion. In the beam axis direction, ray tracing is performed for each beamlet within a treatment field, and the WEPL values of the beamlets are expanded both proximally and distally by the effects of each of these uncertainties. Laterally the CTV is expanded in the same manner as is done traditionally to achieve PTV. Treatment planning then may proceed with range compensator design and dose calculation for each scattered or uniform scanning proton beam, or beam spot weight optimization for pencil beam scanning beam such that each beam delivers a uniform dose distribution conforming to the bsPTV.

With the increasing interests in IMPT applications, especially in treatment of tumors associated with significant organ motion such as lung tumor (28), various methods have been proposed to address the dosimetric effect of organ motion. Specifically, the interplay effect of organ motion relative to the spot-by-spot and layer-by-layer delivery of pencil beam proton therapy treatments has been a subject of intense research efforts (29,30). Similar to conventional photon-based radiotherapy (31), the lateral motion of treatment target in the BEV of a proton treatment field causes the delivery of a pencil beam dose to a voxel of the tumor other than the planned voxel. In addition, state-of-the-art IMPT treatments are delivered in a layer-by-layer manner, where the deepest layer is treated to the prescribed dose, followed by energy changes (range pullback) to deliver prescribed doses to shallow layers of the tumor. Tumor motion in the beam axis direction, as well as organ motion along the beam passage, may cause WEPL changes that result in doses deposited at incorrect layers of tumor or normal tissues. Simulated dose calculation studies have demonstrated dose delivery error of 34% in a single fraction (32) and 18% in 30 fractions (33) for lung treatments; and of 33% in a given fraction for liver (34). Various methods have been proposed to mitigate the dosimetric effects of organ motion in radiotherapy (29,35), including gated therapy; breath-hold; tumor-tracking; and abdominal compression. In addition, IMPT treatments may be delivered via a “repainting” technique (33,36), in which the entire volume is treated multiple times within a treatment fraction, each delivering a portion of the fractional prescribed dose. While all motion mitigation methods will potentially serve to minimize the dosimetric effect of organ motion in proton therapy treatments, they all also require additional verification of organ motion magnitudes and patterns for a given treatment fraction, so as to ensure that deviations of organ motion patterns from their assumed ones at time of patient simulation do not lead to significant dose delivery errors. Dose repainting of the target volume, at 5-10 times in a given fraction, is general considered adequate to minimize the effect of any residual motion modeling error (33,36). This strategy however also may be associated with significantly increased treatment delivery time: at a nominal average dose rate of 2 Gy/min for IMPT treatments, repainting n times would potentially increase the fractional treatment time by n times longer.

Dosimetric effect of patient anatomy variations

Tumor volume changes during course of radiotherapy treatment are common for H&N and lung cancers, and may result in increased doses to critical organs and decreased target dose coverage (37). Adaptive therapy techniques, where off-line repeat patient imaging and re-planning is performed periodically during a patient’s treatment course, are used to minimize the effect of such target volume changes. For proton therapy, these volume changes may significantly change the WEPL of treatment beams, causing even larger dosimetric deviations than would happen for photon-based radiotherapy techniques. Shi et al. (38,39) reported the case of a non-small-cell lung cancer patient, for whom the tumors shrank by up to 80% in volume through the course of a 75.6 Gy (RBE) proton therapy treatment, delivered in 42 daily fractions. Without adaptive re-planning, the patient would receive a lung V20 value 20% higher than originally planned; 150% higher for spinal cord; and 200% higher for esophagus. Adaptive proton therapy for treatment of cancers where the target is subject to shrinkage during the course of treatment is therefore a required component of robust proton therapy practice.

Evaluation of tumor response during radiation therapy course remains an active area of investigations. Currently there are no specific guidelines on the frequency and technique of adaptive re-planning for proton lung cancer treatments. Hui et al. (40) reported results of weekly repeat 4DCT imaging for 8 lung cancer patients with Stage III non-small cell lung cancer receiving IMRT treatments. Proton treatment planning studies showed a mean 4% increase of contra-lateral lung dose; and a mean 4.4 Gy (RBE) dose increase to the spinal cord. Koay et al. (41) reviewed the need for and results of adaptive re-planning of proton therapy for lung cancer. Of the 44 patients enrolled in their clinical trial proton therapy treatment [74 Gy (RBE) in 37 fractions] of stage III non-small cell lung cancer, 9 patients required re-planning due to tumor volume changes that were identified in repeat patient CT imaging, performed at 3-4 weeks after start of treatment. The adaptive re-planning maintained sparing of critical organs such as the esophagus and spinal cord, and prevented inadequate target coverage that would have occurred without re-planning. Beltran et al. (42) reported results of a study of tumor volume changes in craniopharyngioma patients. An average of 6 MRI studies was performed for these patients during their course of radiation therapy treatments. Maximum tumor volume changes ranged between –20.7% and 82%, with a mean of 28.5%. The dosimetric effects of these tumor volume changes were investigated in a subsequent study (43) of 14 similar patients. Comparisons of IMRT, scattered proton therapy, and IMPT treatment plans show higher sensitivity of IMPT than the other two techniques to tumor volume changes. The authors suggest that IMPT re-planning should be considered with a 5% change in PTV volume, while 10% and 25% changes would necessitate re-planning for IMRT and scattered proton therapy respectively.

Patient anatomy changes that impact proton therapy dose delivery accuracy may occur in all tissues along proton beam paths. Albertini et al. (44) reported the dosimetric effect of weight changes for two patients with para-spinal tumors. Treatments were planned and delivered using IMPT technique. Patient weight changes (gaining 1.5 kg for one patient, and losing 8 kg for another) caused WEPL changes along treatment beams, and resulted in optimized beam range changes of +8 mm and –13 mm. It was noted that maximum dose to the cauda equina, the critical organ of concern in the treatments, increased by only 2%, although as much as 80% dose differences were observed within the treated volume locally, due to the range changes. Similarly, changes of stomach and bowel contents in abdominal tumor treatments such as retroperitoneal sarcomas (45), pancreas (46), and liver tumors, may significantly alter the WEPLs of treatment beams. For beams that have to traverse through such organs with potential content changes, alternative CT image datasets are created with the CT numbers of these organs overridden by their potential values for contents that may be present alternatively. Verification plans are then calculated on the overridden image sets to estimate the extent of dosimetric uncertainties due to these organ filling changes. The initial treatment plan may be modified by increasing the proximal and distal margins of the offending treatment field. In general, multiple beams, sometimes non-coplanar, are used, with a majority of target doses delivered via beams having smaller uncertainties, if no other choices are available. Periodic repeat CT or MRI imaging sessions are also used to verify the validity of these verification plans. For prostate treatments, the rectum content may be controlled by filling the rectum with saline or saline-filled rectal balloons, to reduce the impact of daily variability of rectal filling (47).

Relative biological effectiveness (RBE)

Clinically, an RBE value of 1.10 has been assigned to protons of all beam energies, at all depths (11). The RBE of a given proton beam, however, increases significantly toward the end of its beam range (48-55). Current clinical treatment planning systems do not represent the effect of such RBE increases, and it is therefore left to the treatment planning personnel to interpret and include such effects implicitly. In particular, the increase of RBE at the distal end of a proton beam, estimated at up to an additional 25% (over the 1.10 value currently used clinically), also manifests itself in an increase of the beam range (defined to be at the distal 90% of the depth dose) when corrected for RBE by 2 mm or greater (56). For beams that stop before a critical organ, this implies that an additional distal margin of 2 mm or above would need to be included in treatment planning for the offending beam, so that the potential effect of the increased biological dose to the critical organ is approximated in the dose distribution.

Conclusions

Accurate and safe delivery of proton therapy must take into consideration the various uncertainties associated with the calculation and delivery of proton doses. In particular, these include the uncertainties of CT number to relative stopping power ratio conversion, as well as the effect of setup error and organ motion on delivered beam ranges. For traditional scattered beam treatments, addition of distal and proximal margins, as well as use of range compensator smearing margins, has proven adequate for a large variety of tumor treatments. The specific effects of these uncertainties on intensity-modulated proton therapy, however, remain an active area of research. Delivery of IMPT treatments to targets with significant organ motion, therefore, must be done with utmost care.

Acknowledgments

Funding: None.

Footnote

Provenance and Peer Review: This article was commissioned by the Guest Editors (Huan Giap and Eric Y Chuang) for the series “Particle Beam Therapy I” published in Translational Cancer Research. The article has undergone external peer review.

Conflicts of Interest: The author has completed the ICMJE uniform disclosure form (available at http://dx.doi.org/10.3978/j.issn.2218-676X.2012.10.01). The series “Particle Beam Therapy I” was commissioned by the editorial office without any funding or sponsorship. The author has no other conflicts of interest to declare.

Ethical Statement: The author is accountable for all aspects of the work in ensuring that questions related to the accuracy or integrity of any part of the work are appropriately investigated and resolved.

Open Access Statement: This is an Open Access article distributed in accordance with the Creative Commons Attribution-NonCommercial-NoDerivs 4.0 International License (CC BY-NC-ND 4.0), which permits the non-commercial replication and distribution of the article with the strict proviso that no changes or edits are made and the original work is properly cited (including links to both the formal publication through the relevant DOI and the license). See: https://creativecommons.org/licenses/by-nc-nd/4.0/.

References

1. Schneider U, Pedroni E, Lomax A. The calibration of CT Hounsfield units for radiotherapy treatment planning. Phys Med Biol 1996;41:111-24. [PubMed]
2. Yohannes I, Kolditz D, Langner O, et al. A formulation of tissue- and water-equivalent materials using the stoichiometric analysis method for CT-number calibration in radiotherapy treatment planning. Phys Med Biol 2012;57:1173-90. [PubMed]
3. Schaffner B, Pedroni E. The precision of proton range calculations in proton radiotherapy treatment planning: experimental verification of the relation between CT-HU and proton stopping power. Phys Med Biol 1998;43:1579-92. [PubMed]
4. Moyers MF, Miller DW, Bush DA, et al. Methodologies and tools for proton beam design for lung tumors. Int J Radiat Oncol Biol Phys 2001;49:1429-38. [PubMed]
5. Yang M, Zhu XR, Park PC, et al. Comprehensive analysis of proton range uncertainties related to patient stopping-power-ratio estimation using the stoichiometric calibration. Phys Med Biol 2012;57:4095-115. [PubMed]
6. Chang JY, Komaki R, Wen HY, et al. Toxicity and patterns of failure of adaptive/ablative proton therapy for early-stage, medically inoperable non-small cell lung cancer. Int J Radiat Oncol Biol Phys 2011;80:1350-7. [PubMed]
7. Albertini F, Hug EB, Lomax AJ. Is it necessary to plan with safety margins for actively scanned proton therapy? Phys Med Biol 2011;56:4399-413. [PubMed]
8. Lomax AJ, Boehringer T, Coray A, et al. Intensity modulated proton therapy: a clinical example. Med Phys 2001;28:317-24. [PubMed]
9. Inaniwa T, Kanematsu N, Furukawa T, et al. A robust algorithm of intensity modulated proton therapy for critical tissue sparing and target coverage. Phys Med Biol 2011;56:4749-70. [PubMed]
10. Liu W, Zhang X, Li Y, et al. Robust optimization of intensity modulated proton therapy. Med Phys 2012;39:1079-91. [PubMed]
11. International Commission on Radiation Units and Measurements. ICRU Report 78: Prescribing, recording, and reporting proton-beam therapy. J ICRU 2007;7:210.
12. Hong L, Goitein M, Bucciolini M, et al. A pencil beam algorithm for proton dose calculations. Phys Med Biol 1996;41:1305-30. [PubMed]
13. Schaffner B, Pedroni E, Lomax A. Dose calculation models for proton treatment planning using a dynamic beam delivery system: an attempt to include density heterogeneity effects in the analytical dose calculation. Phys Med Biol 1999;44:27-41. [PubMed]
14. Wagner MS. Automated range compensation for proton therapy. Med Phys 1982;9:749-52. [PubMed]
15. Urie M, Goitein M, Wagner M. Compensating for heterogeneities in proton radiation therapy. Phys Med Biol 1984;29:553-66. [PubMed]
16. Li G. Experimental comparison of range compensator calculation algorithms and milling strategies. 51st Particle Therapy Collaborative Group Annual Meeting 2012.
17. Hugo G, Vargas C, Liang J, et al. Changes in the respiratory pattern during radiotherapy for cancer in the lung. Radiother Oncol 2006;78:326-31. [PubMed]
18. Trofimov A, Nguyen PL, Efstathiou JA, et al. Interfractional variations in the setup of pelvic bony anatomy and soft tissue, and their implications on the delivery of proton therapy for localized prostate cancer. Int J Radiat Oncol Biol Phys 2011;80:928-37. [PubMed]
19. Kang Y, Zhang X, Chang JY, et al. 4D Proton treatment planning strategy for mobile lung tumors. Int J Radiat Oncol Biol Phys 2007;67:906-14. [PubMed]
20. Engelsman M, Rietzel E, Kooy HM. Four-dimensional proton treatment planning for lung tumors. Int J Radiat Oncol Biol Phys 2006;64:1589-95. [PubMed]
21. Engelsman M, Kooy HM. Target volume dose considerations in proton beam treatment planning for lung tumors. Med Phys 2005;32:3549-57. [PubMed]
22. Bert C, Rietzel E. 4D treatment planning for scanned ion beams. Radiat Oncol 2007;2:24. [PubMed]
23. Knopf A, Bert C, Heath E, et al. Special report: workshop on 4D-treatment planning in actively scanned particle therapy--recommendations, technical challenges, and future research directions. Med Phys 2010;37:4608-14. [PubMed]
24. Morávek Z, Rickhey M, Hartmann M, et al. Uncertainty reduction in intensity modulated proton therapy by inverse Monte Carlo treatment planning. Phys Med Biol 2009;54:4803-19. [PubMed]
25. Shirato H, Onimaru R, Ishikawa M, et al. Real-time 4-D radiotherapy for lung cancer. Cancer Sci 2012;103:1-6. [PubMed]
26. Unkelbach J, Bortfeld T, Martin BC, et al. Reducing the sensitivity of IMPT treatment plans to setup errors and range uncertainties via probabilistic treatment planning. Med Phys 2009;36:149-63. [PubMed]
27. Park PC, Zhu XR, Lee AK, et al. A beam-specific planning target volume (PTV) design for proton therapy to account for setup and range uncertainties. Int J Radiat Oncol Biol Phys 2012;82:e329-36. [PubMed]
28. Chang JY, Cox JD. Improving radiation conformality in the treatment of non-small cell lung cancer. Semin Radiat Oncol 2010;20:171-7. [PubMed]
29. Bert C, Durante M. Motion in radiotherapy: particle therapy. Phys Med Biol 2011;56:R113-44. [PubMed]
30. Lomax AJ. Intensity modulated proton therapy and its sensitivity to treatment uncertainties 2: the potential effects of inter-fraction and inter-field motions. Phys Med Biol 2008;53:1043-56. [PubMed]
31. Yu CX, Jaffray DA, Wong JW. The effects of intra-fraction organ motion on the delivery of dynamic intensity modulation. Phys Med Biol 1998;43:91-104. [PubMed]
32. Kraus KM, Heath E, Oelfke U. Dosimetric consequences of tumour motion due to respiration for a scanned proton beam. Phys Med Biol 2011;56:6563-81. [PubMed]
33. Seco J, Robertson D, Trofimov A, et al. Breathing interplay effects during proton beam scanning: simulation and statistical analysis. Phys Med Biol 2009;54:N283-94.
34. Zhang Y, Boye D, Tanner C, et al. Respiratory liver motion estimation and its effect on scanned proton beam therapy. Phys Med Biol 2012;57:1779-95. [PubMed]
35. Keall PJ, Mageras GS, Balter JM, et al. The management of respiratory motion in radiation oncology report of AAPM Task Group 76. Med Phys 2006;33:3874-900. [PubMed]
36. Zenklusen SM, Pedroni E, Meer D. A study on repainting strategies for treating moderately moving targets with proton pencil beam scanning at the new Gantry 2 at PSI. Phys Med Biol 2010;55:5103-21. [PubMed]
37. Barker JL Jr, Garden AS, Ang KK, et al. Quantification of volumetric and geometric changes occurring during fractionated radiotherapy for head-and-neck cancer using an integrated CT/linear accelerator system. Int J Radiat Oncol Biol Phys 2004;59:960-70. [PubMed]
38. Shi W, Nichols RC, Flampouri S, et al. Tumour shrinkage during proton-based chemoradiation for non-small-cell lung cancer may necessitate adaptive replanning during treatment. Hong Kong J Radiol 2011;14:190-4.
39. Shi W, Nichols RC, Flampouri S, et al. Proton-based chemoradiation for synchronous bilateral non-small-cell lung cancers: a case report. Thoracic Cancer 2012. [Epub ahead of print].
40. Hui Z, Zhang X, Starkschall G, et al. Effects of interfractional motion and anatomic changes on proton therapy dose distribution in lung cancer. Int J Radiat Oncol Biol Phys 2008;72:1385-95. [PubMed]
41. Koay EJ, Lege D, Mohan R, et al. Adaptive/nonadaptive proton radiation planning and outcomes in a phase II trial for locally advanced non-small cell lung cancer. Int J Radiat Oncol Biol Phys 2012;84:1093-100. [PubMed]
42. Beltran C, Naik M, Merchant TE. Dosimetric effect of target expansion and setup uncertainty during radiation therapy in pediatric craniopharyngioma. Radiother Oncol 2010;97:399-403. [PubMed]
43. Beltran C, Roca M, Merchant TE. On the benefits and risks of proton therapy in pediatric craniopharyngioma. Int J Radiat Oncol Biol Phys 2012;82:e281-7. [PubMed]
44. Albertini F, Bolsi A, Lomax AJ, et al. Sensitivity of intensity modulated proton therapy plans to changes in patient weight. Radiother Oncol 2008;86:187-94. [PubMed]
45. Swanson EL, Indelicato DJ, Louis D, et al. Comparison of three-dimensional (3D) conformal proton radiotherapy (RT), 3D conformal photon RT, and intensity-modulated RT for retroperitoneal and intra-abdominal sarcomas. Int J Radiat Oncol Biol Phys 2012;83:1549-57. [PubMed]
46. Nichols RC Jr, Huh SN, Prado KL, et al. Protons offer reduced normal-tissue exposure for patients receiving postoperative radiotherapy for resected pancreatic head cancer. Int J Radiat Oncol Biol Phys 2012;83:158-63. [PubMed]
47. Vargas C, Mahajan C, Fryer A, et al. Rectal dose-volume differences using proton radiotherapy and a rectal balloon or water alone for the treatment of prostate cancer. Int J Radiat Oncol Biol Phys 2007;69:1110-6. [PubMed]
48. Paganetti H, Niemierko A, Ancukiewicz M, et al. Relative biological effectiveness (RBE) values for proton beam therapy. Int J Radiat Oncol Biol Phys 2002;53:407-21. [PubMed]
49. Paganetti H. Significance and implementation of RBE variations in proton beam therapy. Technol Cancer Res Treat 2003;2:413-26. [PubMed]
50. Wilkens JJ, Oelfke U. Optimization of radiobiological effects in intensity modulated proton therapy. Med Phys 2005;32:455-65. [PubMed]
51. Tilly N, Johansson J, Isacsson U, et al. The influence of RBE variations in a clinical proton treatment plan for a hypopharynx cancer. Phys Med Biol 2005;50:2765-77. [PubMed]
52. Wambersie A, Hendry JH, Andreo P, et al. The RBE issues in ion-beam therapy: conclusions of a joint IAEA/ICRU working group regarding quantities and units. Radiat Prot Dosimetry 2006;122:463-70. [PubMed]
53. Jäkel O. The relative biological effectiveness of proton and ion beams. Z Med Phys 2008;18:276-85. [PubMed]
54. Frese MC, Wilkens JJ, Huber PE, et al. Application of constant vs. variable relative biological effectiveness in treatment planning of intensity-modulated proton therapy. Int J Radiat Oncol Biol Phys 2011;79:80-8. [PubMed]
55. Wambersie A, Menzel HG, Andreo P, et al. Isoeffective dose: a concept for biological weighting of absorbed dose in proton and heavier-ion therapies. Radiat Prot Dosimetry 2011;143:481-6. [PubMed]
56. Carabe A, Moteabbed M, Depauw N, et al. Range uncertainty in proton therapy due to variable biological effectiveness. Phys Med Biol 2012;57:1159-72. [PubMed]